讲座人: 纪友清教授、朱森教授
讲座时间：15:00
讲座日期：2020-11-09
地点：腾讯会议（ID：401750651 ）
主办单位：数学与信息科学学院
报告题目一： Power Set of Some Quasinilpotent weighted shifts
报告人: 纪友清教授
报告人简介：
纪友清，吉林大学教授，博士生导师，长期从事算子理论与算子代数研究，主持多项国家自然科学基金项目及教育部高等学校博士点专项基金等项目，在Trans. Amer. Math.、 J. Funct. Anal.、J.Operator Theory等国内外期刊上发表了重要学术论文。2004年入选教育部新世纪优秀人才支持计划。
讲座简介：
For a quasinilpotent operator T, write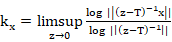 
for each nonzero vector x. Set 
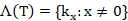$, and call it the power set of T. This notation was introduced by Douglas and Yang. They showed thatfor 
, 
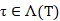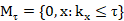$ is a linear subspace invariant under each A commuting with T; hence, if there are two different points 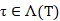such that 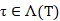are closed, then T has a nontrivial hyperinvariant subspace. It is natural to consider the following questions. Which subsets can be the power set of a quasinilpotent operator? Is 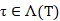closed? I will talk something about
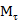and the closeness of.
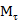报告题目二：随机Toeplitz代数
报告人: 朱森教授
讲座时间：16:30
报告人简介：
朱森，吉林大学数学学院教授，博士生导师。主持国家自然科学基金青年、面上等项目。近年来主要从事线性算子的复对称性、随机理论等方面的研究，在 J. Funct. Anal., J. London Math. Soc., Math. Ann., Trans. AMS 等杂志发表系列论文。
讲座简介：
给定独立同分布的随机变量{X_n}_{n\geq 1}, 我们以T表示以{X_n}为权的随机Hardy移位，其生成的C*代数我们称为与T相关的随机Toeplitz代数。本报告将介绍我们关于这一C*代数的若干初步结果，包括理想、表示、稳定秩等。这些结果是经典Toeplitz代数相关结果的随机版本。
